
During the 4th century B.C., Greek geometry burst its bonds and went on to the tremendous discoveries of the "age of giants." And Greek culture, too, burst from the mainland of Hellas and spread to most of the eastern Mediterranean.
Both developments were connected with the romantic figure of Alexander the Great. After Plato's time, teachers and alumni from the Academy had gone on to found schools of their own. In particular, Plato's most famous associate, the great philosopher Aristotle, had set up the Lyceum in Athens, and started the systematic classification of human knowledge. And Aristotle's most renowned pupil was the warrior king Alexander of Macedon, who tried to conquer the world.
In thirteen years, Alexander extended his rule over Greece proper, and Ionia, Phoenicia, Egypt, and the vast Persian domains as far as India. Then he died, and his empire broke up. But throughout those far-flung lands, he had founded Greek cities and planted the seeds of Greek civilization-the Greek language, Greek art, and, of course, Greek mathematics.
Mathematicians traveled with his armies. And there is even a
Menaechmus, who had studied with Plato and Eudoxus, was trying to teach Alexander some geometric proofs. The lesson went badly. "Master," exclaimed Alexander, "in my kingdom there are royal roads built smoothly, as short cuts for the king. Can you not make this task easier for me?"
Menaechmus made the now famous reply, "Sire, there is no royal road to geometry."
Yet there was to be a royal road, after all. And before and after Menaechmus, many geometers labored mightily to build it.
During the 5th and 4th centuries B.C., these geometers held the Greek belief that abstract thought, not mechanical work, was the proper occupation for a gentleman. So they kept aloof from practical applications, and devoted themselves to smoothing and polishing existing proofs and finding more and more new ones. These thinkers were of various kinds-dreamers, adventurers, discoverers, organizers. Some fitted into one or another of these categories, some belonged to all of them. We shall only call the roll of the most important. Among the earlier ones were Archytas of Tarentum, Plato's geometry teacher, Hippocrates of Chios, who tried to fit together all the rules, and Theodorus of Cyrene, who discovered many of the irrationals. In Plato's own time, the two greatest were Theaetetus of Athens and Eudoxus of Cnidus. And at the Lyceum, and at the Academy after Plato, many more worked to improve the definitions and assumptions and make new abstract discoveries-including Menaechmus, Alexander's taskmaster.
But after Alexander's conquests, the antimechanical taboo was lifted. A new atmosphere prevailed in the Hellenistic, or part
And mathematicians turned their attention to practical matters. They made water clocks, irrigation devices, cogs for launching ships, various sorts of tackles and gears.
The most illustrious mathematician of this later age was also the mechanical wizard of antiquity, Archimedes of Syracuse. Among his many inventions was the so-called "screw of Archimedes," a device for pumping water from the holds of leaky ships or draining flooded mines.
So vast was his knowledge of leverage that he once said, "Give me a place to stand on, and I will move the earth!"

On one occasion the Roman ships were burned by fireballs hurled from a catapult behind the city walls.
Another time, a large claw maneuvered by levers and pulleys actually grabbed the prows of the Roman ships, raised them in the air, and flung them back into the sea.
At another attack, a huge mirror concentrated the sun's rays and focused them on the Roman fleet, setting ships on fire.
Naturally, at each suggestion of a return to Syracuse, the unhappy Roman sailors would cry, "Oh no! Not again!" And Marcellus himself used to call Archimedes "that geometrical hundred-armed giant."
Archimedes' many machines proved the value of science in war and peace even at that early date. Yet he did much more important and far-reaching work in pure mathematics. lie and the other Alexandrian geometers were every bit as devoted to abstract thought as their predecessors. In fact, from the Golden Age through the Age of Plato and right into the Hellenistic Age, they were all absorbed in a set of fascinating abstract questions.
These were the three famous puzzles-constructions that had to he performed using only straightedge and string. One was called 'squaring the circle": how could you construct a square with the same area as a circle? Another was "trisecting an angle":
how could you divide air angle into three equal parts? Another was doubling the cube": how could you construct a cube whose volume would be double that of another cube?
Historians do not think this tale is true. Rather, they believe, it was made up later to hide the fact that the "three geometric puzzles" were really useless problems. But working on what may seem useless has frequently been the task of mathematicians, and such tasks, pursued with care, patience, and persistence, have led to most useful results. A whole book could be written about useful results from useless problems.
In the case of the three geometric puzzles, they were not only useless but quite impossible with only those tools. These constructions simply cannot be made with string and straightedge alone! But more than two thousand years elapsed before that was definitely proved.
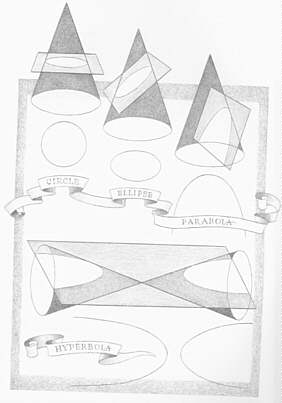
Menaechmus himself, struggling with the problem of doubling the cube, dreamed up the idea of cutting a cone with a plane. The sections he cut were an epochal discovery, and later on Archimedes worked on them himself.
They were in the shape of three new curves, the ellipse, the parabola, and the hyperbola-the Creek names of that age are still in use today, and we still call them conic sections, because they can be cut from a cone.
If you cut a cone of your own, you can see these curves for yourself. Take an ordinary ice-cream cone-not a real one, as it would crumble if you cut it, but the shape of one. Set the vertex up; this is a right circular cone. If you cut straight across it, parallel to the base, you will get a circle which is also a conic section. Slice clear across it at an angle, and you get an ellipse. Now, just slice off one side of the cone, cutting parallel to the slanting outside of the cone itself (the line of generation), and you get a parabola. Finally, slice straight down parallel to the vertical axis of the cone-and stand another cone upside down on top of the first one and slice it in the same way-and you get the two curves of a hyperbola. Picture the sides of your cone extending endlessly, and you will see the open arms of the parabola and the hyperbola reaching out toward infinity. These three curves-the ellipse, the parabola, and the hyperbola-are a perfect example of remarkable results from a mathematical puzzle.
But it took two thousand years for the conics to be recognized for what they were: the paths of motion for all bodies, celestial and earthly alike.
It wasn't until the 17th century that the great astronomer Kepler discovered his famous law that the path of each planet around the sun is actually an ellipse. In the same century, Galileo proved that a cannon ball or any other missile shot into the air will travel a path that is a parabola. Less than a century later, Newton developed his universal laws of motion and his great law of gravitation. Without the Conica of Apollonius, which he knew thoroughly, it is unthinkable that Newton could
And today, twenty-three centuries after Archimedes studied them, you can see the conics everywhere, for they have countless uses in science and industry.
Every time you throw a ball, the ball's path traces a parabola. When a jet of water rises from a fountain, it describes a parabola as it falls back into the pool beneath. The path of Astronaut Shepard's trip into outer space and back was a parabola. And this curve has characteristics that make it valuable for reflecting light and sound waves. That is why parabolic reflectors are used in searchlights, flashlights, car headlights, and in radar antennas and radio telescopes.
So the conic sections-found by Menaechmus from a "useless" puzzle-have proved tremendously useful to later science and industry. And so have the great practical achievements of the Alexandrian geometers.
Archimedes' work on levers and floating bodies was the beginning of the science of mechanics. And his method of determining curved areas and volumes was the forerunner of Newton's calculus. In fact, both the theoretical and the applied mathematics of the Hellenistic Age prepared the way for the massive achievements of Sir Isaac Newton, who laid the foundations of the modern exact sciences. As Newton himself said, "I could not have seen so far, had I not stood on the shoulders of giants."
So Menaechmus was partly wrong, after all, that there was no royal road to geometry. For the giants of Greek geometry did build a royal road that others would tread after two thousand years.
And this "age of giants" was to produce a pinnacle of its own.