

Our story of ancient geometry reaches its thrilling climax at the start of the 3rd century B.C., for then a famous geometer wrote down the whole theoretical subject in the best-selling mathematics text of all time, and soon after, another geometer performed the most spectacular practical feat. He used a shadow to measure not just a pyramid, but the whole round earth!
These two events took place in the view Greek capital of the age-old land of Egypt.
Founded by Alexander the Great and named for him, Alexandria had become the leading metropolis of the ancient world. By now, it was a flourishing royal city, a beehive of commerce, the most important seaport on the whole Mediterranean. And Alexandria was also the world's main clearing-house for ideas.
This sumptuous cosmopolitan city was the gathering place for the best scholars and scientists of the age. Savants from many lands made their discoveries in the "museum"- a graduate school that carried on studies in literature, medicine, astronomy, and
The librarian was a Creek named Eratosthenes. A universal mind, he was a mathematician, a specialist in history, an astronomer, and a poet besides. And around 250 B.C. he did something almost incredible in those times. Eratosthenes measured accurately the girth of the planet he lived on!
Strange as it seems, he had a practical purpose in mind. As a great geographer, he understood that the earth was round, and he was mapping the known parts of it. On his map of the world, Eratosthenes put all the data and distances he could get. The project was typical of that era, when the Mediterranean was becoming "one world" for the first time. It was one world of scientists; astronomers, mathematicians, and geographers in many lands were pooling their knowledge. And it was one world of trade, of ships and sailors, who needed maps. To make his map more accurate and useful, Eratosthenes wanted to determine the width of a degree of latitude. But for that, he had to know the circumference of the earth. How was he to measure it?
The inspiration came to him one day as he was traveling up the Nile on a summer study trip. He noticed with excitement that on the longest day of the year the noonday sun shone straight down a well at Syene, a town about 5000 stadia up the river from Alexandria. He could see the shape of the sun reflected on the surface of the water at the bottom of the well. But from there northward to Alexandria where he lived, the sun never got directly overhead;
So Syene was really on the Tropic of Cancer! To a geographer that was most important, and he explored the region in order to draw the Tropic on his map.
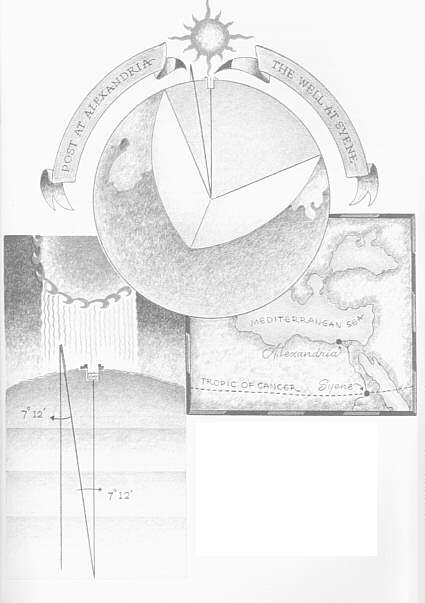
But the sight of the sun in the well fired his imagination even more. Just that single observation, plus his knowledge of geometry and his own active brain, told him how to determine the distance around the earth. He did it by means of a shadow and some remarkably shrewd deductions. Eratosthenes simply took the known distance between Syene and Alexandria, due north-as reported by camel caravans and professional "step- counters" -and then measured a single angle at the right place and the right time.
He made his historic measurement at Alexandria, at noontime on the longest day of the year. At that moment, he knew, the sun was shining straight down the well at Syene, 5000 stadia away, casting no shadow. But at Alexandria, where he stood, an upright post was casting a shadow.
So Eratosthenes stretched a string from the top of the post to the tip of its shadow. Then he measured the angle between the post (at its top) and the string. Do you see what he had done? The string represented the sun's ray that was casting the shadow.
He had measured the angle at which the post met the sun's ray: It was a 7* 12' angle.
Now look at the picture where we have cut away a piece of the earth, and you can see the brilliant deduction that Eratosthenes made from this angle.
erat1
He just assumed an imaginary line from the sun rays at Syene continuing straight down through the well to the center of the earth, and an imaginary line from the post at Alexandria continuing down to the center of the earth, too. Of course, he assumed the sun's rays were parallel. So at the center of the earth, the post line met the parallel ray line at the same angle that the post met the ray at Alexandria! (When a transversal cuts
This angle goes into 360* just 50 times. (There are 60 minutes to the degree.) Then, since the length of the arc made by 7*12' was about 5000 stadia, the distance around the whole earth must be 50 times that, or about 250,000 stadia.
In Egypt there were about 10 stadia to a mile, so his measurement was around 25,000 miles-very close to the actual circumference of the earth, as it was measured in later centuries.
Eratosthenes' estimate was the most accurate in ancient times, and the climactic feat of the ancient practical art of geometry, or earth measurement. Yet his contemporaries thought of him as a second-string man," for around the same time, and in the same city of Alexandria, there lived another geometer whose name is more widely known than any mathematician's in history.
Surely you have guessed who it was. None other than Euclid himself! His masterpiece, the Elements, became world famous in his own lifetime, and nowadays it is still just as famous. For more than two thousand years, ever since he wrote it, students have been studying elementary geometry from his great work. The chances are that it is the basis of your geometry book today.
The incidents of Euclid's life are unknown. But we can infer the traits of painstaking accuracy, imagination, dogged determination, amid above all logical thinking, that led him to assemble and organize everything that had been accomplished in geometry up to his time.