
Thales the "father of geometry," was a sort of Greek Benjamin Franklin. The known facts of his life are few. He was a merchant. He traveled extensively to the older centers of civilization and learned much on his travels. He said ''the magnet has a soul because it moves the iron," showing he had studied lodestones. And he is believed to have been the first to experiment with electricity, the static kind in a piece of rubbed amber.

But Thales was also a picturesque character and inspired some of the choicest of Aesop's "Fables." Many stories of his accomplishments were told by later writers, some serious and some quite fanciful. True or not, these tales teach us much about Thales' way of thought. He was forever asking "Why?" and working out his answer from what he saw, and standing ready to prove it. Even the anecdotes about his business ventures show this, especially the amusing tale of Thales and the oil presses.
One afternoon as Thales and his friends were discussing money-coins had just recently been invented-Thales made the remark, "Anybody can make money if he puts his mind to it."
His friends immediately said, "Prove it."
Thales was in an awkward spot and he had to think and think. He said to himself, "What item is useful to everybody?" His answer was, "Oil."
In 600 B.C. oil didn't mean petroleum, but olive oil. Olive oil was used for soap. It provided fuel for lamps. It was used for cooking. And it was prized as a skin-softener.
Thales decided to study oil from the tree to the oil press. During this investigation, the first stumbling block he found was the fact that for several seasons the trees had not been producing olives. Why? Thales thought next about weather conditions. In fact, he had to do research on the weather of past seasons-the kind favorable to the ripening of the olive, the kind unfavorable.
After that, he also had to try to discover a pattern in the weather conditions, so that he could see what to expect in the future. From his diligent work in laying out the pattern from the past, he calculated that favorable weather conditions were due the next season.
But when the big crop came the following year, there were no presses to borrow and none to buy either-Thales had bought them all. So Thales cornered the oil market and made a fortune. Some say he gave the presses back afterwards, because he didn't have time to go into the oil business.
Anyhow, the anecdote shows how his mind worked. He was a great observer. He wotild study the pattern of repeated occurrences and then prophesy the natural path.
Another famous story, told by Aesop, illustrates the same mental traits. It shows that Thales was not above trying to follow the reasoning of a little donkey.
Thales had inherited a salt mine The salt was transported from the mines by donkeys. They were weighed down with bags of salt at the deposits, and then had to carry them to the market. This donkey train had a long journey in the hot sun.
As they crossed a stream en route, one little donkey was so warm and fatigued that he just collapsed in the cool water and rolled over. Afterward he not only felt refreshed for the rest of the trip, but realized that a great weight had been removed from his back. On every trip thereafter, he repeated this same stunt.
His master Thales was surprised at the beast's fresh appearance, disappointed in his scant cargo, and very puzzled as to how it had been dissolved. For a while the donkey outsmarted Thales, but in the long run Thales paid him back by using some simple deductive reasoning. Thales asked himself, "What sort
of thing refreshes the donkey and dissolves the salt? . . . A cool stream. . . . Is there a stream along the route? . Yes! . . . What will absorb the water and fatigue the donkey? . . . Sponges!" So on the next trip Thales filled the saddle bags with sponges instead of salt, and the little donkey's happy habit was broken.
So as a businessman in lonia, Thales was already using a new type of thinking. But two other interests led him to establish the science of geometry: his travels in the Near East, and his study of shadows.
A story is told about how he took up both.
As he was walking in his garden one night, enraptured by the sparkling splendor of the stars, suddenly the silent stillness of the night was broken by the sound of a great splash and a gurgle. Thales had stepped majestically into a well!

Nobody likes to be damp and laughed at. In the days that followed, Thales decided to look at the hot dry earth beneath his feet. He would study the shadow patterns that lay there, speaking so eloquently of the sun's messages upon the earth! And he would see more of the earth itself, by traveling to the ancient countries of Mesopotamia and Egypt. (From what we know of Thales, we can guess that he probably decided to engage in some shipping and foreign trade on the side.)
The first stop on his journey was Babylon, a glamorous city with a long history and a large library of cuneiform tablets. There he was fascinated by the impressive records of the stargazers. He stayed for quite a while-poring over the charts, studying the methods of sky measurement, learning the use of the circle and its divisions for measuring angles and directions.
Then he crossed over into Egypt. In that land he mastered the construiction of engineering works. He studied the irrigation canals, the well-laid-out fields, the wall decorations showing the history of Egypt in pictures, the designs in Egyptian decorations.
He was absorbing all the old practical geometry of Egypt and Mesopotamia. This was typically Greek. In those times, Greece herself was busily learning from the older civilizations.
And he brought to his travels another trait that was typical of the Greeks and the future civilization they were building. He had a new kind of inquiring mind.
Everywhere he went, Thales studied the shadows traced on these flat ancient lands by ziggurats, obelisks, buildings, and people. He saw these shadows as men had never seen them

Here was a remarkable traveler, a Greek Benjamin Franklin indeed! If we can believe the tales of Thales at home and abroad, he took with him his fresh lonian insight, even as he absorbed the old practical lore of the Babylonians and Egyptians. Out of this combination was to come the new theoretical geometry.
In the Land of the Nile-so the legend goes-Thales amazed and frightened his guides by telling them, as if by magic, the exact height of the Great Pyramid.
The story is worth reviewing in some detail. It shows us Thales' new geometry in action, and enables us to compare it with the old Egyptian kind.
Naturally, Thales' visit to Egypt was not complete without a sightseeing trip to the desert at Giza, to see the three pyramids and the Sphinx half-buried in the sand nearby. In 6oo B.C. the pyramids were about 2000 years old.
Thales engaged guides and took a Greek friend along. When they reached those mighty monuments, the guides seemed proud to boast that the Egyptian pyramids had been standing when the ancestors of the Creeks were "long-haired barbarians."
Thales stood for a time admiring the most gigantic of the tombs: the Great Pyramid of Cheops, which covers more than twelve acres! He looked tip the great slope, rising to a peak against the cloudless Egyptian sky, and noticed how the brilliant sunlight hit directly against one face and drew a pointed shadow over the desert sands. Then he asked his celebrated question.
"How high is this pyramid?"
The guides were dumbfounded and got into a lengthy discussion. No sightseer had ever asked them that before. Visitors were always content with the dimensions of the pyramid's square base-252 paces along each side. Sometimes the Creek tourists didn't believe that, and had to pace it off for themselves. But
While the argument went on, Thales and his friend had been walking around quietly, staying close to the pyramid's shadow, where it was cool. Suddenly the Greeks hallooed.
"Never mind my question!" called Thales, as the guides approached. "I know the answer. The Great Pyramid at Giza rises to a height of 160 paces!"
Terrified, the guides flung themselves on their faces before Thales, fully convinced that he was a magician.
To be sure, Thales did not get the answer by magic. he simply measured two shadows on the sand, and then used an abstract rule from his new kind of geometry.
To show you just what his method was-and the way he probably worked it out, and how different it was from the old geometry of the pyramid builders-we shall go back and imagine a preliminary scene.
When Thales reached Egypt to spend the winter as tourist and student and merchant traveler, he must have had many things to do. Perhaps there was business to transact on the crowded streets and wharves. Of course he wanted to see the famous monuments, the colossal statues and pyramid tombs.
Now his studies were over, and he was going to start sightseeing.
It was a warm sunny afternoon, and he was sitting outside the temple, waiting to bid farewell to the high priest Thothmes, a most important man. The attendant would take quite a while to fetch him.
As he waited, Thales studied the scene and thought about the accomplishments of Egyptian geometry. In the great empty space before the temple stood a high gilded obelisk, making a fine "shadow pole," or sundial, in the afternoon sunlight. A few white- clad priests and worshipers were standing about, their shadows very distinct, too. Off to one side was the vast temple, perfectly laid out so that its sides would face the four points of the compass. And through its colonnade he could just glimpse a great wall painting, a masterpiece of Egyptian proportion.
Proportion . . . It ran, he knew, like a golden thread all through the work of the ancients. Proportion was used by the Chaldean astronomers in the angles of their sextants, and the corresponding arcs of the travels of distant stars. Proportion was used by Egyptian architects, in designing their buildings and erecting the actual structures. But it was best shown in the vast wall paintings with which the Egyptians decorated their temples and tombs. Those vivid scenes were all painted by artists using an innate feeling for proportion.
He had seen them at work. The Egyptian artist had a very

That was intuitive proportion and most practical, the Egyptians at their best. . . .
But as Thales sat there, watching the shadows lengthen outside the temple, he saw something entirely different: abstract proportion.
But Thales saw far more than that. He saw the motion of the lengthening shadows. Surely others had seen it too, as they sat waiting, but he saw it with an "X-ray eye."
For as Thales watched, he noticed something truly remarkable. All tile shadows changed together, in length and direction. At first, they were all half as long as the objects that cast them. Later, they were all the same length as the objects. Later still, the shadows were all twice as long as the height of the objects.
Probably many men had observed something like that, over the centuries. But the lonian traveler tried to find a constant pattern. He had to prove it was always so, and to find out why.
And he did!
Thales noticed that all the abstract right triangles changed together, too-not the whole triangles. The right angle and the height of the object that made its upright or vertical side-these did not change. But the rest of the triangle changed as the sun seemed to change its position in the sky. The sun was so far away that its rays hit the tops of all the objects, and the tips of all the shadows, at the same slant. So, as the sun was higher or lower, the other two angles had to change in all the triangles. And as the angles changed, the other two sides had to change too-the length of the shadow (the flat or horizontal base of the triangle), and the length of the sun's ray from top to tip (the slanting third side).
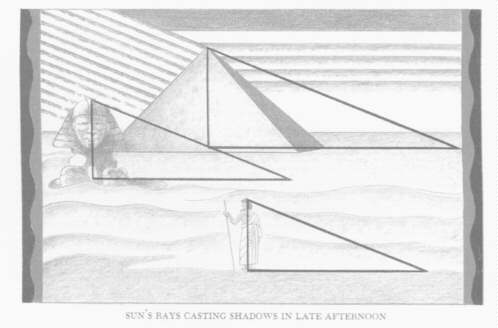

So at each moment, all the sun-made righttriangles were exactly the same shape-not the same size, but the same shape: the right angles and heights of the objects remained unchanged, hut the other two sides and other two angles changed as the sun seemed to move across the sky.
Now Thales knew his eyes hadn't deceived him. The shadow lengths would always change together in the same way, while the heights of the objects must of course stay unchanged. he had his secret for measuring the height of the pyramid.
Before you hear exactly how Thales accomplished that feat, you may want to try out his secret for yourself.
You can watch these same shadow changes on your own playground or ball field by comparing the right triangles formed by the flagpole, the basketball backboard, and your own height.
Start, say, in mid-afternoon when your shadow is as long as you are tall. At that same time, the flagpole's shadow will be as long as the flagpole is high. And the shadow of the basketball backboard will also be equal to its height. So you can pace off the shadows of the flagpole and the basketball backboard to find their heights, without bothering to climb up with a measuring tape.
Of course, you could start earlier in the afternoon, at a time when your shadow is about half of your height. Then you could pace off the other two shadows, double their length, amid so find the height of the other objects.
Or you might wait till later in the day, when your shadow is twice as long as your height. To be sure, the other shadows would also be twice the height of their objects. So you could pace off the other two shadows, and take half of the distance-and that would give you the height of the flagpole or the backboard.
Getting it is easy. The "secret, " you already know, is simphy a proportion. As Thales did, you will always find that at any moment there is a constant relation between one object's height and its shadow, and the next object's height and its shadow. In this case, you are using the equal ratios between the height of an object and its shadow, and your height and your shadow.
Just put it like this:
HEIGHT OF OBJECT (Ho) is to SHADOW OF OBJECT (So) as Your Height (Hy) is to Your Shadow (Sy).
You can write this as equal ratios, Ho:So: :Hy:Sy, or as an equation of fractions,

Then simply clear the first fraction (multiply both sides of the equation by So), and you get
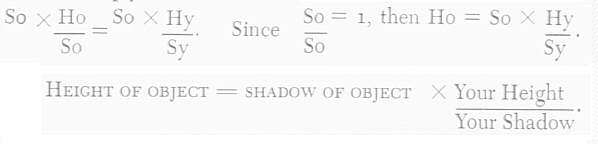
Now that you know the secret for yourself, you will want to see (in your imagination) exactly how Thales measured the height of the pyramid.
When he asked his famous question, the guides, you remember, began to talk and argue. Meantime Thales, who already
So he made his calculation as shown in the illustration.
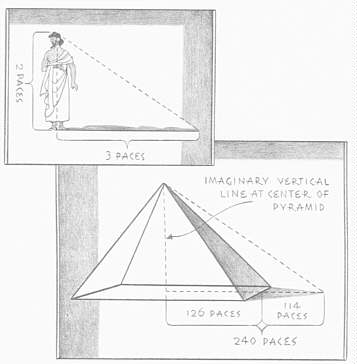
Do you see what Thales did? He used an abstract right triangle! He pictured the height of the Great Pyramid as an imaginary post from its top straight down to its base. Such an imaginary post would cast an imaginary shadow, all the way from where it stood at the center of the pyramid clear out to the tip of the pyramid's real shadow: so the length of this imaginary shadow would be one-half the length of the base plus the actual projecting shadow! Therefore:

Of course, the guides promptly spread the news of Thales magical solution to this seemingly impossible problem. When the priests of Thoth verified that 160 paces was indeed the height of the Great Pyramid, according to the old records, popular astonishment knew no bounds.
The tale traveled far and wide, so far and wide that it has come down to us after 2500 years. And the story has even more meaning today.
For the Great Pyramid was a sturdy monument to ancient practical geometry. But Thales' shadow-reckoning of its height was an even more stalwart monument in the development of reasoning.